Fine-tuning a BERT model for search applications

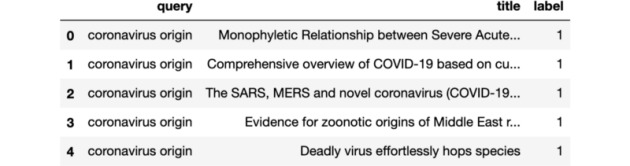
How to ensure training and serving encoding compatibility

There are cases where the inputs to your Transformer model are pairs of sentences, but you want to process each sentence of the pair at different times due to your application’s nature. Search applications are one example.

Photo by Alice Dietrich on Unsplash

The search use case

Search applications involve a large collection of documents that can be pre-processed and stored before a search action is required. On the other hand, a query triggers a search action, and we can only process it in real-time. Search apps’ goal is to return the most relevant documents to the query as quickly as possible. By applying the tokenizer to the documents as soon as we feed them to the application, we only need to tokenize the query when a search action is required, saving us precious time.

In addition to applying the tokenizer at different times, you also want to retain adequate control about encoding your pair of sentences. For search, you might want to have a joint input vector of length 128 where the query, which is usually smaller than the document, contributes with 32 tokens while the document can take up to 96 tokens.

Training and serving compatibility

When training a Transformer model for search, you want to ensure that the training data will follow the same pattern used by the search engine serving the final model. I have written a blog post on how to get started with BERT model fine-tuning using the transformer library. This piece will adapt the training routine with a custom encoding based on two separate tokenizers to reproduce how a Vespa application would serve the model once deployed.

Create independent BERT encodings

The only change required is simple but essential. In my previous post, we discussed the vanilla case where we applied the tokenizer directly to the pairs of queries and documents.

from transformers import BertTokenizerFast

model_name = "google/bert_uncased_L-4_H-512_A-8"
tokenizer = BertTokenizerFast.from_pretrained(model_name)

train_encodings = tokenizer(
    train_queries, train_docs, truncation=True, 
    padding='max_length', max_length=128
)
val_encodings = tokenizer(
    val_queries, val_docs, truncation=True, 
    padding='max_length', max_length=128
)

In the search case, we create the create_bert_encodings function that will apply two different tokenizers, one for the query and the other for the document. In addition to allowing for different query and document max_length, we also need to set add_special_tokens=False and not use padding as those need to be included by our custom code when joining the tokens generated by the tokenizer.

def create_bert_encodings(
    queries, docs, tokenizer, query_input_size, doc_input_size
):
    queries_encodings = tokenizer(
        queries, truncation=True, 
        max_length=query_input_size-2, add_special_tokens=False
    )
    docs_encodings = tokenizer(
        docs, truncation=True, 
        max_length=doc_input_size-1, add_special_tokens=False
    )

    TOKEN_NONE=0
    TOKEN_CLS=101
    TOKEN_SEP=102

    input_ids = []
    token_type_ids = []
    attention_mask = []
    for query_input_ids, doc_input_ids in zip(
        queries_encodings["input_ids"], docs_encodings["input_ids"]
    ):
        # create input id
        input_id = [TOKEN_CLS] + query_input_ids + [TOKEN_SEP] + 
            doc_input_ids + [TOKEN_SEP]
        number_tokens = len(input_id)
        padding_length = max(128 - number_tokens, 0)
        input_id = input_id + [TOKEN_NONE] * padding_length
        input_ids.append(input_id)
        # create token id
        token_type_id = [0] * 
            len([TOKEN_CLS] + query_input_ids + [TOKEN_SEP]) + 
            [1] * len(doc_input_ids + [TOKEN_SEP]) + 
            [TOKEN_NONE] * padding_length
        token_type_ids.append(token_type_id)
        # create attention_mask
        attention_mask.append(
            [1] * number_tokens + [TOKEN_NONE] * padding_length
        )

    encodings = {
        "input_ids": input_ids,
        "token_type_ids": token_type_ids,
        "attention_mask": attention_mask
    }
    return encodings

We then create the train_encodings and val_encodings required by the training routine. Everything else on the training routine works just the same.

from transformers import BertTokenizerFast

model_name = "google/bert_uncased_L-4_H-512_A-8"
tokenizer = BertTokenizerFast.from_pretrained(model_name)

train_encodings = create_bert_encodings(
    queries=train_queries, 
    docs=train_docs, 
    tokenizer=tokenizer, 
    query_input_size=32, 
    doc_input_size=96
)

val_encodings = create_bert_encodings(
    queries=val_queries, 
    docs=val_docs, 
    tokenizer=tokenizer, 
    query_input_size=32, 
    doc_input_size=96
)

Conclusion and future work

Training a model to deploy in a search application require us to ensure that the training encodings are compatible with encodings used at serving time. We generate document encodings offline when feeding the documents to the search engine while creating query encoding at run-time upon arrival of the query. It is often relevant to use different maximum lengths for queries and documents, and other possible configurations.

Photo by Steve Johnson on Unsplash

We showed how to customize BERT model encodings to ensure this training and serving compatibility. However, a better approach is to build tools that bridge the gap between training and serving by allowing users to request training data that respects by default the encodings used when serving the model. pyvespa will include such integration to make it easier for Vespa users to train BERT models without having to adjust the encoding generation manually as we did above.

Fine-tuning a BERT model with transformers

Setup a custom Dataset, fine-tune BERT with Transformers Trainer, and export the model via ONNX

This post describes a simple way to get started with fine-tuning transformer models. It will cover the basics and introduce you to the amazing Trainer class from the transformers library. You can run the code from Google Colab but do not forget to enable GPU support.

Photo by Samule Sun on Unsplash

We use a dataset built from COVID-19 Open Research Dataset Challenge. This work is one small piece of a larger project that is to build the cord19 search app.

Install required libraries

!pip install pandas transformers

Load the dataset

To fine-tune the BERT models for the cord19 application, we need to generate a set of query-document features and labels that indicate which documents are relevant for the specific queries. For this exercise, we will use the query string to represent the query and the title string to represent the documents.

training_data = read_csv("https://thigm85.github.io/data/cord19/cord19-query-title-label.csv")
training_data.head()

There are 50 unique queries.

len(training_data["query"].unique())

50

For each query, we have a list of documents, divided between relevant (label=1) and irrelevant (label=0).

training_data[["title", "label"]].groupby("label").count()

Data split

We are going to use a simple data split into train and validation sets for illustration purposes. Even though we have more than 50 thousand data points when considering unique query and document pairs, I believe this specific case would benefit from cross-validation since it has only 50 queries containing relevance judgment.

from sklearn.model_selection import train_test_split
train_queries, val_queries, train_docs, val_docs, train_labels, val_labels = train_test_split(
    training_data["query"].tolist(), 
    training_data["title"].tolist(), 
    training_data["label"].tolist(), 
    test_size=.2
)

Create BERT encodings

Create a train and validation encodings. To do that, we need to chose which BERT model to use. We will use padding and truncation because the training routine expects all tensors within a batch to have the same dimensions.

from transformers import BertTokenizerFast

model_name = "google/bert_uncased_L-4_H-512_A-8"
tokenizer = BertTokenizerFast.from_pretrained(model_name)

train_encodings = tokenizer(train_queries, train_docs, truncation=True, padding='max_length', max_length=128)
val_encodings = tokenizer(val_queries, val_docs, truncation=True, padding='max_length', max_length=128)

Create a custom dataset

Now that we have the encodings and the labels, we can create a Dataset object as described in the transformers webpage about custom datasets.

import torch

class Cord19Dataset(torch.utils.data.Dataset):
    def __init__(self, encodings, labels):
        self.encodings = encodings
        self.labels = labels

    def __getitem__(self, idx):
        item = {key: torch.tensor(val[idx]) for key, val in self.encodings.items()}
        item['labels'] = torch.tensor(self.labels[idx])
        return item

    def __len__(self):
        return len(self.labels)

train_dataset = Cord19Dataset(train_encodings, train_labels)
val_dataset = Cord19Dataset(val_encodings, val_labels)

Fine-tune the BERT model

We are going to use BertForSequenceClassification, since we are trying to classify query and document pairs into two distinct classes (non-relevant, relevant).

from transformers import BertForSequenceClassification

model = BertForSequenceClassification.from_pretrained(model_name)

We can set requires_grad to False for all the base model parameters to fine-tune only the task-specific parameters.

for param in model.base_model.parameters():
    param.requires_grad = False

We can then fine-tune the model with Trainer. Below is a basic routine with an out-of-the-box set of parameters. Care should be taken when choosing the parameters below, but this is out of this piece’s scope.

from transformers import Trainer, TrainingArguments

training_args = TrainingArguments(
    output_dir='./results',          # output directory
    evaluation_strategy="epoch",     # Evaluation is done at the end of each epoch.
    num_train_epochs=3,              # total number of training epochs
    per_device_train_batch_size=16,  # batch size per device during training
    per_device_eval_batch_size=64,   # batch size for evaluation
    warmup_steps=500,                # number of warmup steps for learning rate scheduler
    weight_decay=0.01,               # strength of weight decay
    save_total_limit=1,              # limit the total amount of checkpoints. Deletes the older checkpoints.    
)


trainer = Trainer(
    model=model,                         # the instantiated 🤗 Transformers model to be trained
    args=training_args,                  # training arguments, defined above
    train_dataset=train_dataset,         # training dataset
    eval_dataset=val_dataset             # evaluation dataset
)

trainer.train()

Export the model to ONNX

Once training is complete, we can export the model using the ONNX format to be deployed elsewhere. I assume below that you have access to a GPU, which you can get from Google Colab, for example.

from torch.onnx import export

device = torch.device("cuda") 

model_onnx_path = "model.onnx"
dummy_input = (
    train_dataset[0]["input_ids"].unsqueeze(0).to(device), 
    train_dataset[0]["token_type_ids"].unsqueeze(0).to(device), 
    train_dataset[0]["attention_mask"].unsqueeze(0).to(device)
)
input_names = ["input_ids", "token_type_ids", "attention_mask"]
output_names = ["logits"]
export(
    model, dummy_input, model_onnx_path, input_names = input_names, 
    output_names = output_names, verbose=False, opset_version=11
)

Concluding remarks

As mentioned before, this post covered basic training setup. This is a good starting point to be improved upon. It is better to start simple and complement than the opposite, especially when learning something new. I left important topics such as hyperparameter tuning, cross-validation, and more detailed model validation to followup posts. But having a basic training setup is a good first step.

Weakly informative priors for logistic regression

On a previous post, I have mentioned what is called the separation problem [1]. It can happen for example in a logistic regression, when a predictor (or combination of predictors) can perfectly predicts (separate) the data, leading to infinite Maximum Likelihood Estimate (MLE) due to a flat likelihood.

I also mentioned that one (possibly) naive solution to the problem could be to blindly exclude the predictors responsible for the problem. Other more elegant solutions include a penalized likelihood approach [1] and the use of weakly informative priors [2]. In this post, I would like to discuss the latter.

Model setup

Our model of interest here is a simple logistic regression

and since we are talking about Bayesian statistics the only thing left to complete our model specification is to assign prior distributions to ‘s. If you are not used to the above notation take a look here to see logistic regression from a more (non-Bayesian) Machine Learning oriented viewpoint.

Weakly informative priors

The idea proposed by Andrew Gelman and co-authors in [2] is to use minimal generic prior knowledge, enough to regularize the extreme inference that are obtained from maximum likelihood estimation. More specifically, they realized that we rarely encounter situations where a typical change in an input corresponds to the probability of the outcome changing from 0.01 to 0.99. Hence, we are willing to assign a prior distribution to the coefficient associated with that gives low probability to changes of 10 on logistic scale.

After some experimentation they settled with a Cauchy prior with scale parameter equal to (Figure above) for the coefficients , . When combined with pre-processed inputs with standard deviation equal to 0.5, this implies that the absolute difference in logit probability should be less then 5, when moving from one standard deviation below the mean, to one standard deviation above the mean, in any input variable. A Cauchy prior with scale parameter equal to was proposed for the intercept . The difference is because if we use a Cauchy with scale for it would mean that would probably be between and for units that are average for all inputs and as a default prior this might be too strong assumption. With scale equal to 10, is probably within and in such a case.

There is also a nice (and important) discussion about the pre-processing of input variables in [2] that I will keep for a future post.

Conclusion

I am in favor of the idea behind weakly informative priors. If we have some sensible information about the problem at hand we should find a way to encode it in our models. And Bayesian statistics provides an ideal framework for such a task. In the particular case of the separation problem in logistic regression, it was able to avoid the infinite estimates obtained with MLE and give sensible solutions to a variety of problems just by adding sensible generic information relevant to logistic regression.

References:

[1] Zorn, C. (2005). A solution to separation in binary response models. Political Analysis, 13(2), 157-170.
[2] Gelman, A., Jakulin, A., Pittau, M.G. and Su, Y.S. (2008). A weakly informative default prior distribution for logistic and other regression models. The Annals of Applied Statistics, 1360-1383.